Rate law and stoichiometry
Chemical Reaction Engineering
Rate law
Reactor mole balance in terms of X
| Reactor | Mole balance |
|---|---|
| Batch | N_{A0}\frac{dX}{dt} = r_AV |
| CSTR | V = \frac{F_{A0}X}{-r_A} |
| PFR | F_{A0}\frac{dX}{dV} = -r_A |
| PBR | F_{A0}\frac{dX}{dW} = -r'_A |
Rate law
The algebraic expression for the reaction rate equation, r_j.
-r_A = f \left[ \begin{array}{c} \text{temperature} \\ \text{dependent} \\ \text{terms} \end{array} , \begin{array}{c} \text{concentration} \\ \text{dependent} \\ \text{terms} \end{array} \right] = f(T, C)]
How to derive an equation for –r_A in terms of X_A
Relate all r_j to C_j \Rightarrow Rate law
Relate all C_j to V or \upsilon \Rightarrow Stoichiometry
Relate V or \upsilon to X_A \Rightarrow Stoichiometry
Put together
r_A = f(T, X_A)
Basic definitions
Homogeneous reaction: involves only one phase.
Heterogeneous reaction: involves more than one phase. Reaction usually occurs at the interface.
Irreversible reaction: proceeds in only one direction. Continues until one of the reactants is exhausted.
Reversible reaction: proceeds in either direction. Continues until equilibrium concentration is reached.
No reaction is completely irreversible. For irreversible reactions equilibrium point lies far to the product side.
Reaction rate
Rate laws: are the algebraic equations that apply to a given reaction
Relative rates: how fast one species appears or disappears relative to the other species in a given reaction.
Net rate of formation of a given species (e.g., A): is the sum of the rate of the reactions of A for all the reactions in which A is either a reactant or product.
Molecularity of reaction
Number of atoms, ions, or molecules involved (colliding) in a reaction step
Unimolecular: One molecule colliding in one reaction step
radioactive decay
alpha decay of Uranium-238 emitting an alpha particle (a helium nucleus) and formation of Thorium-234.
\ce{ _{92}U^{238} -> _{90}Th^{234} + _{2}He^{4} }
rate of disappearance of U \Rightarrow -r_U = kC_U
Bimolecular: Two molecule colliding in one reaction step
Only true bimolecular reactions are those involving collision with free radicals
initiation step of the free radical bromination of ethane
\ce{ Br. + C2H6 -> HBr + C2H5.}
-r_{\ce{Br.}} = kC_{\ce{Br.}}C_{\ce{C2H6}}
Trimolecular: Three molecule colliding in one reaction step
The probability of trimolecular reaction is almost non-existent
In most instances the reaction pathway follows a series of bimolecular reactions.
Relative rates of reaction
How fast one species appears or disappears relative to the other species in a given reaction.
Can be obtained from the ratio of stoichiometric coefficients
Consider a reaction
\ce{aA + bB -> cC + dD}
For every mole of A consumed \frac{c}{a} moles of C appear
r_C = \left[ \begin{array}{c} \text{rate of} \\ \text{formation of C}\end{array} \right] = \frac{c}{a} \left[ \begin{array}{c} \text{rate of} \\ \text{disappearance of A}\end{array} \right] = \frac{c}{a} (-r_A)
r_C = \frac{-c}{a} r_A Similarly, r_C = \frac{c}{d} r_D
\begin{align*} \ce{A} & \quad + \quad & \ce{\frac{b}{a} B} &\quad \ce{->} \quad & \ce{\frac{c}{a} C} &\quad + \quad & \ce{\frac{d}{a} D} \\ \frac{-r_A}{a} & \quad = \quad & \frac{-r_B}{b} & \quad = \quad & \frac{r_C}{c} & \quad = \quad & \frac{r_D}{d} \end{align*}
In class exercise: relative rates
- For the reaction \ce{2A + B -> 3C}, calculate rates of reaction of B and C if -r_A = 10 mol/(dm^3 s)
In class exercise: relative rates
- \ce{NO2} is being formed at a rate of 4 mol/(m^3 s) in the reaction \ce{2NO + O2 <=> 2NO2}. Calculate the rate of consumption of \ce{NO}, and \ce{O2}.
Rate law
The rate of reaction depends on collision frequency of the molecules.
Concentration and temperature
Molecular collision frequency \propto concentration
\Rightarrow Rate of reaction \propto concentration. -r_A = f(C_A, C_B, ...) \quad \text{for const. T}
As temperature increases, collision frequency increases
\Rightarrow Rate of reaction \propto temperature. -r_A = f([T],[C_A, C_B, ...])
For many reactions
-r_A = k_A(T) f(C_A, C_B, ...)
Specific rate of reaction (rate constant), k_A(T)
Proportionality constant in the rate equation
Depends on the temperature (following the Arrhenius equation), the presence of a catalyst, and other environmental conditions but is independent of the reactant concentrations.
Power law model
Dependence of -r_A on concentration of species present (f(C_j)) is almost always determined by experimental observations.
Functional dependence on concentration may be postulated by theory
\Rightarrow Experiments are required to confirm the proposed form
Power law model is most common general forms of the rate law
-r_A = k_A C_A^\alpha C_B^\beta
Order of reaction: the powers to which the concentrations are raised
- The reaction is \alpha order with respect to A and \beta order with respect to B.
- Overall order of reaction n = \alpha + \beta.
k_A: Specific reaction rate
\text{Units of } k_A = \frac{\text{(conc)}^{1-n}}{\text{time}}
Order of reaction
\ce{A -> Products}
| Reaction order | Rate equation | Units of k_A |
|---|---|---|
| 0^{th} order | -r_A = k_A | \{k\} = \frac{mol}{volume \ s} |
| 1^{st} order | -r_A = k_A C_A | \{k\} = \frac{1}{s} |
| 2^{nd} order | -r_A = k_A C_A^2 | \{k\} = \frac{volume}{mol \ s} |
| 3^{rd} order | -r_A = k_A C_A^3 | \{k\} = \frac{(volume/mol)^2}{s} |
Elementary reaction
A reaction involving single step is called an elementary reaction.
Stoichiometric coefficients in the reaction are equal to the powers in the rate law.
![]()
\ce{2NO + O2 -> 2NO2}
This reaction is not elementary, but under some conditions it follows an elementary rate law
-r_{NO} = k_{NO} C_{NO}^2 C_{O_2}
\Rightarrow Elementary as written
\ce{CO + Cl2 -> COCl2}
This reaction is non-elementary
-r_{CO} = k_{CO} C_{CO} C_{Cl_2}^{3/2}
1^{st} order with respect to \ce{CO} and 3/2 order with resect to \ce{Cl2} (5/2 order overall)
\Rightarrow Non-elementary reaction
Complex rate expression
\ce{2N2O -> 2N2 + O2} \qquad -r_{N_2O} = \frac{ k_{N_2O} C_{N2O}}{1 + k' C_{O_2}}
Rate expression cannot be separated into solely temperature dependent and concentration dependent terms.
Overall reaction order cannot be stated
Only undrer limiting circumstances we can speak of reaction order
for 1 >> k'C_{O_2}; -r_{N_2O} = k_{N_2O} C_{N_2O} \Rightarrow ‘Apparent’ first order reaction
for 1 << k'C_{O_2}; -r_{N_2O} = k_{N_2O} \frac{C_{N_2O}}{C_{O_2}} \Rightarrow ‘Apparent’ 0^{th} order reaction
Reaction is -1^{th} order with \ce{O2} and 1^{st} order with \ce{N2O}.
This kind of rate expression is common for liquid and gaseous reactions promoted by solid catalysts.
Heterogeneous reactions
Historically for many gas-solid catalyzed reactions it is customary to write rate laws in terms of partial pressures rather than concentrations.
Weight of catalyst is important rather than reactor volume (\Rightarrow -r'_A)
Hydromethylation of toluene
\ce{C6H5CH3 + H2 ->[{cat}] C6H6 + CH4}
-r'_T = \frac{k P_{H_2} P_T}{1 + K_B P_B + K_T P_T}
- ’ (prime): indicates typical units are in /g-cat
- P: partial pressures (kPa or atm)
- K: adsorption constant (1/kPa or atm^{-1})
- [K] = \frac{mol \ toluene}{kg\ cat \cdot s \cdot kPa^2}
Use ideal gas law to express the reaction in terms of concentrations: P_i = C_i RT
Reversible reaction
All rate laws must reduce to thermodynamic relationships relating the reacting species concentrations at equilibrium
At equilibrium rate of reaction is zero for all the species (-r_A \equiv 0)
For a reaction \ce{aA + bB <=> cC + dD}
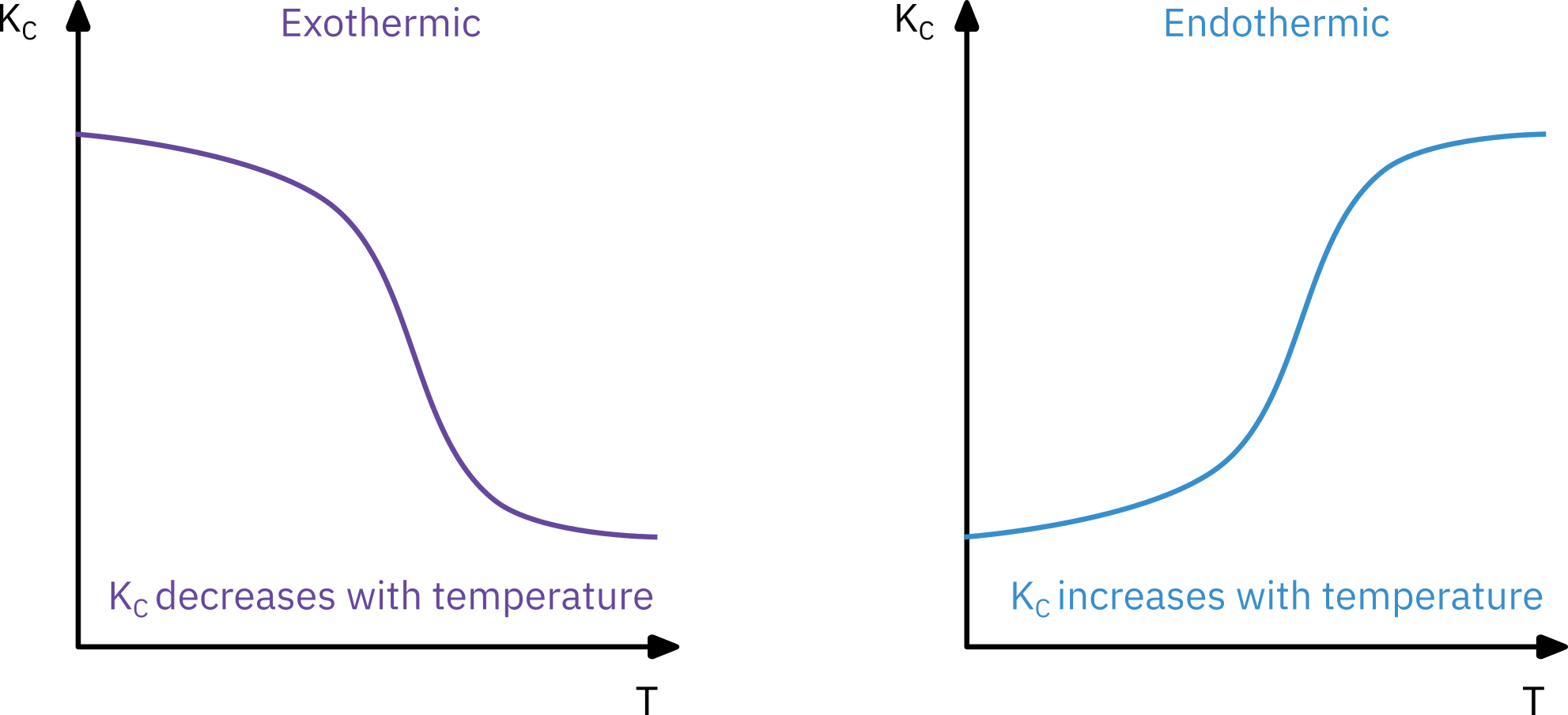
K_c = \frac{C_{C_e}^c C_{D_e}^d}{C_{A_e}^a C_{B_e}^b} \qquad [K_c] = \left(\frac{mol}{volume}\right)^{c +d -a -b}
For elementary reaction \ce{A <=>[{k_f}][{k_r}] B}
-r_A = k \left( C_A - \frac{C_B}{K_c} \right); K_c = \frac{k_f}{k_r}
In class exercise: rate law
Determine the rate law for the reaction described in each of the cases below involving species A, B, and C. The rate laws should be elementary as written for reactions that are either of the form \ce{A -> B} or \ce{A + B -> C}.
- The units of the specific reaction rate are k = \left[\frac{dm^3}{mol \ h} \right].
- The units of the specific reaction rate are k = \left[\frac{mol}{kg-cat \ h \ (atm)^2} \right].
- The units of the specific reaction rate are k = \left[\frac{1}{h} \right].
- The units of a nonelementary reaction rate are k = \left[\frac{mol}{dm^3 \ h} \right].
In class exercise: rate law
For the reaction \ce{C6H6 <=>[{k_B}][{k_{-B}}] C6H4 + H2} (\ce{B <=> D + H2})
determine the rate expression for disappearance of benzene (-r_B). Assume both the forward and reverse reactions are elementary.
Equilibrium constant
van’t Hoff equation
When there is no change in number of moles and heat capacity term \Delta C_p = 0
K_c(T) = K_c(T_1) exp \left[ \frac{\Delta H_{Rx}^\circ}{R} \left( \frac{1}{T_1} - \frac{1}{T} \right)\right]
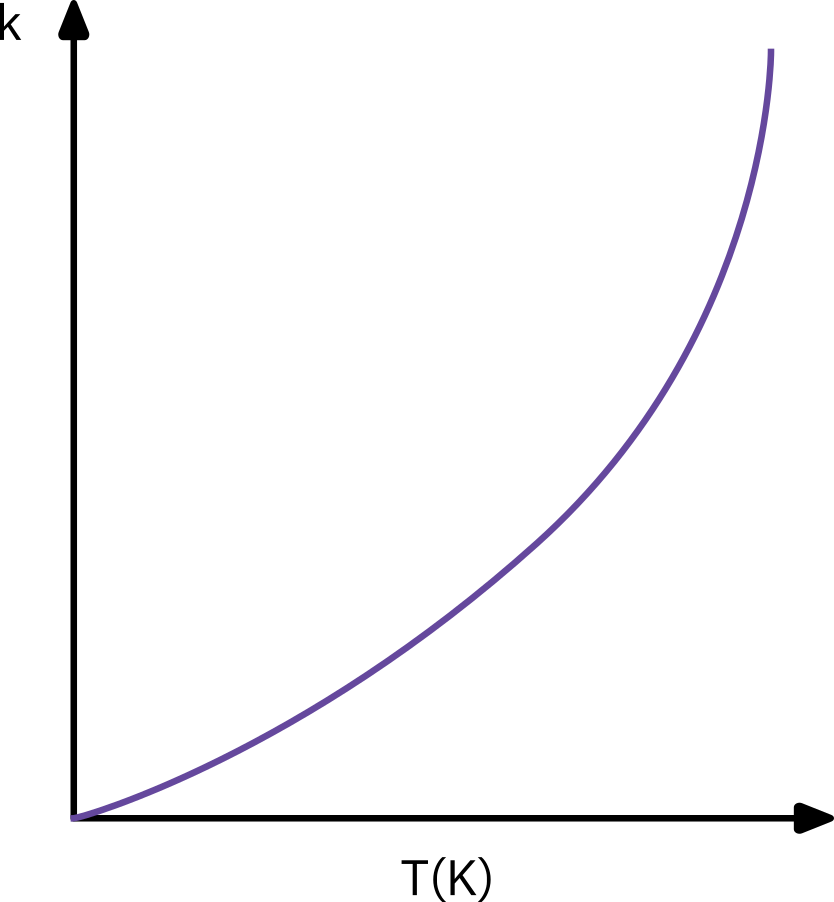
The reaction rate constant (k_A)
\Rightarrow Not a constant. Just independent of concentration
Arrhenius equation
k_A(T) = A e^{\frac{-E}{RT}} \Rightarrow k_0 e^{-E/RT}
- k_0, A: Preexponential factor/ frequency factor
- E: Activation energy (J/mol) or (cal/mol)
- R: Gas constant (= 8.314 J/mol K)
- T: Absolute temperature (K)
Empirically varified over a large temperature range
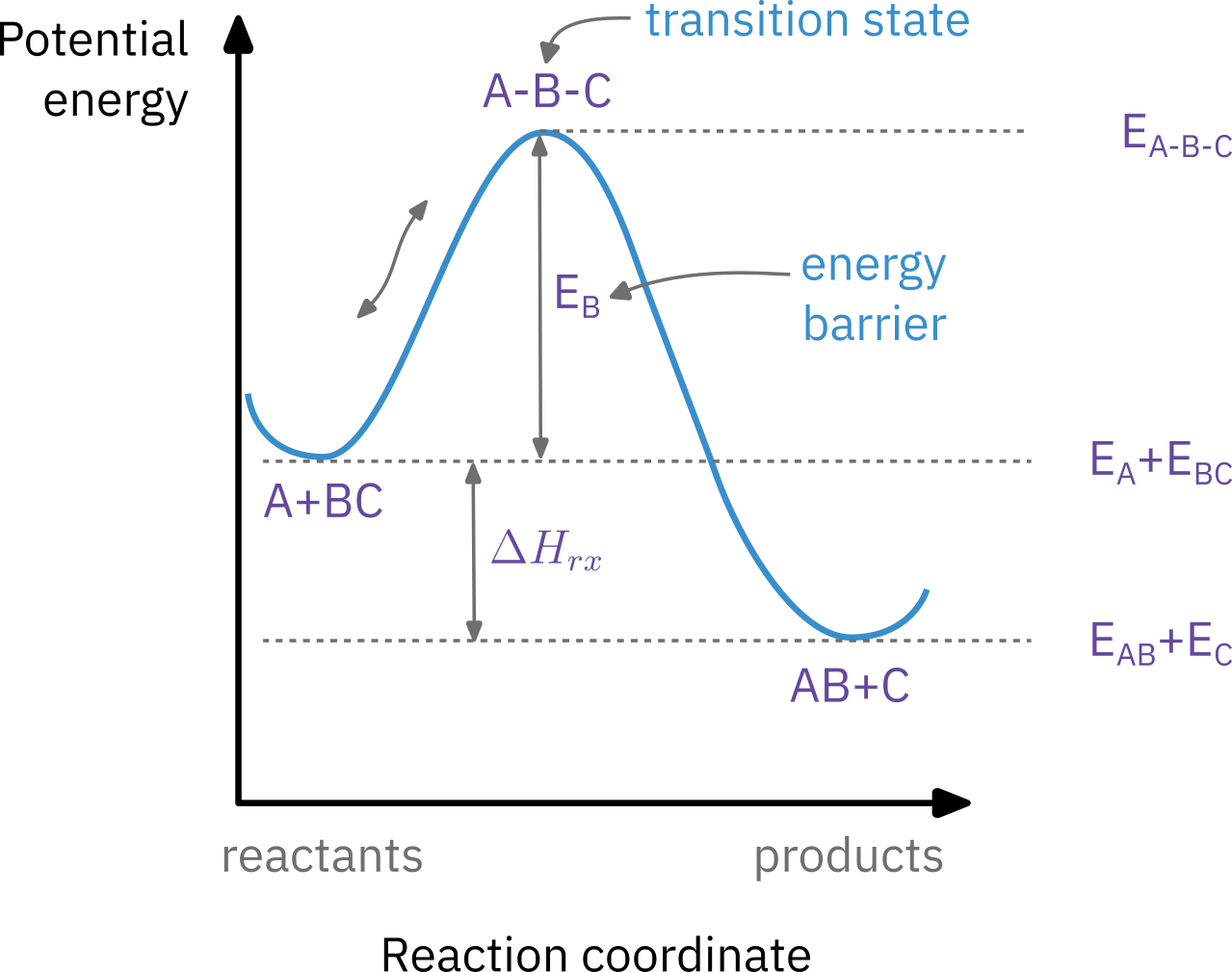
Activation energy
Barrier to energy transfer (from kinetic energy to potential energy) between reacting molecules that must must be overcome.
Minimum increase in potential energy of the reactants that must be provided to transform the reactants into products
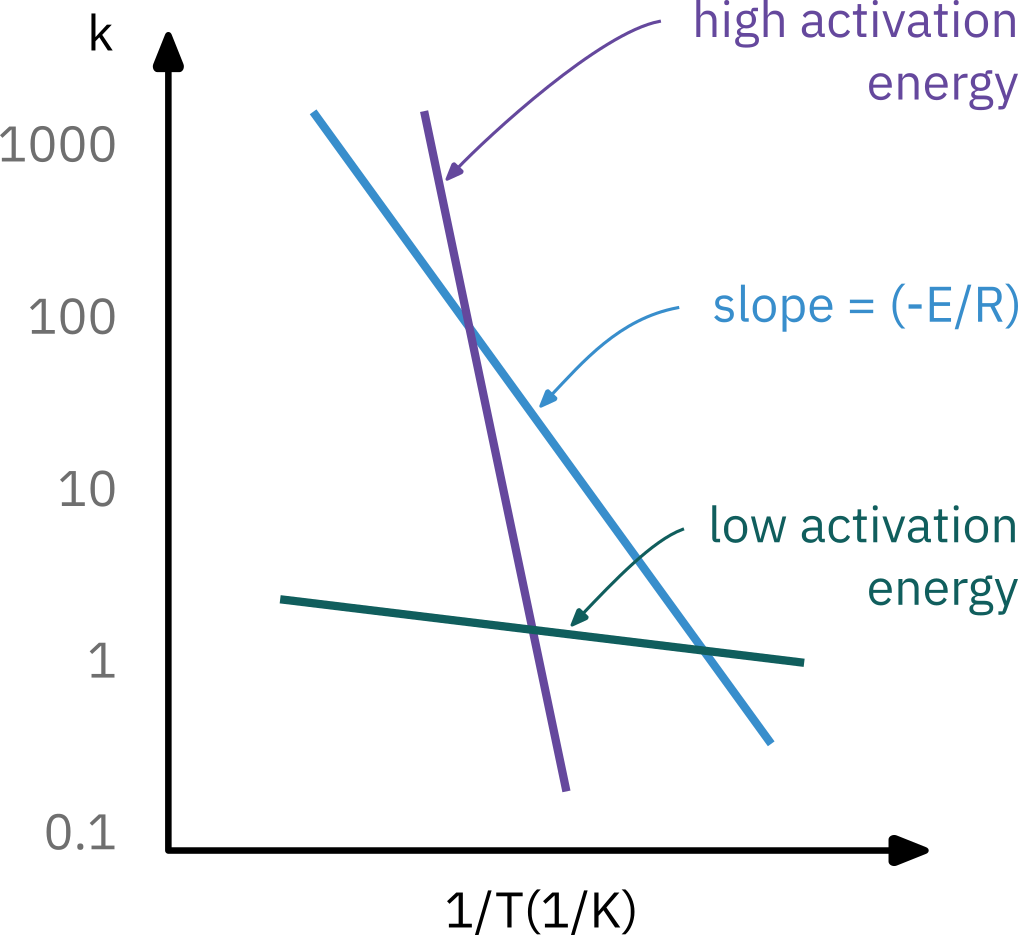
Determining activation energy
Experimentally measuring reaction rate at different temperatures
k_A(T) = A e^{-E/RT}
Taking natural log
\ln k_A = \ln A -\frac{E}{R} \left( \frac{1}{T} \right)
k(T) = k(T_0) e^{\frac{E}{R} \left( \frac{1}{T_0} - \frac{1}{T} \right)}
- Rule of thumb: Reaction rate doubles every 10 °C rise in temperature
Arrhenius plot
In class exercise: activation energy
The decomposition of benzene diazonium chloride to give chlorobenzene and nitrogen
\ce{C6H5N2Cl -> C6H5Cl + N2}
follows first order kinetics. The rate constant data at different temperatures is given in Table 1. Calculate the activation energy.
| k (s^{-1}) | 0.00043 | 0.00103 | 0.00180 | 0.00355 | 0.00717 |
| T (K) | 313.0 | 319.0 | 323.0 | 328.0 | 333.0 |
Stoichiometry
If the rate law depends on more than ne species, we must relate concentrations of different species to each other.
Stoichiometric table
Represents stoichiometric relationships between reacting molecules for a single reaction
How many molecules of one species will be formed during a chemical reaction given a number of molecules of another species disappear.
Let us consider general reaction
\ce{aA +bB -> cC + dD}
relative rates
\frac{-r_A}{a} = \frac{-r_B}{b} = \frac{r_C}{c} = \frac{-r_D}{d}
Basis of calculation: Species A
\ce{A +\frac{b}{a}B -> \frac{c}{a}C + \frac{d}{a}D}
Batch systems
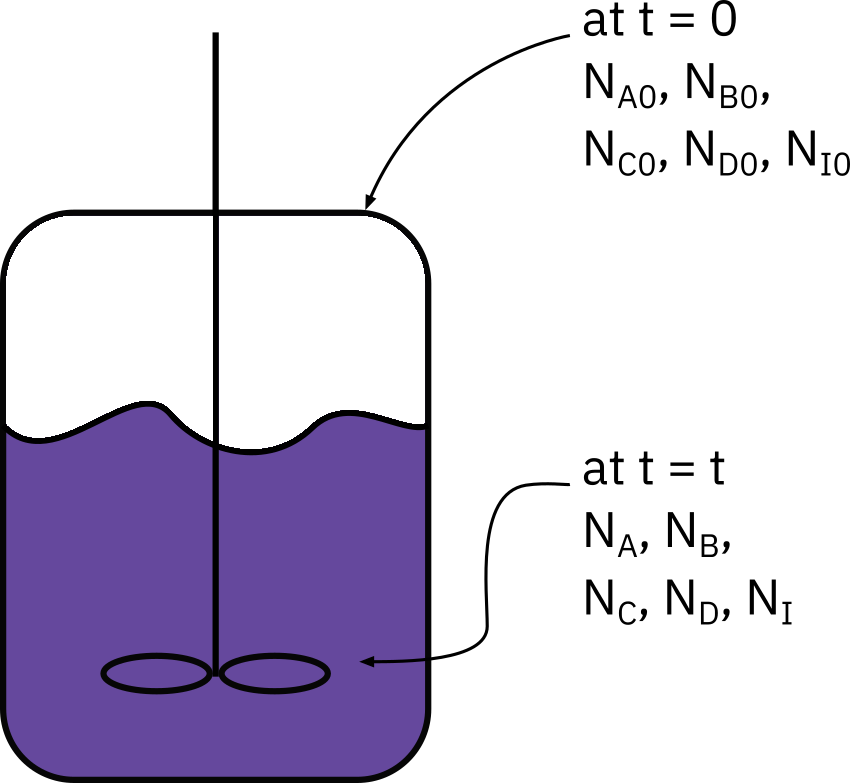
Mole balance
\frac{dN_A}{dt}=r_A V
N_{A0} : No. of moles of A initially present
X : Conversion at time t
N_{A0}X : No. of moles of A consumed at time t
N_{A} : No. of moles of A in system at time t
N_{A} = N_{A0}-N_{A0}X = N_{A0}(1-X)
Stoichiometric table: batch systems
Moles of B reacted
moles\ of\ B\ reacted = \frac{mol\ B\ reacted}{mol\ A\ reacted} \times mol\ A\ reacted = \frac{b}{a} N_{A0} X
Species Initially (mol) Change (mol) Remaining (mol) A N_{A0} -N_{A0}X N_A = N_{A0} - N_{A0}X B N_{B0} -(b/a)N_{A0}X N_B = N_{B0} -(b/a)N_{A0}X C N_{C0} (c/a)N_{A0}X N_C = N_{C0} +(c/a)N_{A0}X D N_{D0} (d/a)N_{A0}X N_D = N_{D0} +(d/a)N_{A0}X I N_{I0} 0 N_I = N_{I0} Total \mathbf{N_{T0}} \mathbf{N_T = N_{T0} + \delta N_{A0}X}
\delta = +\frac{d}{a}+\frac{c}{a}-\frac{b}{a}-1
Change in total number of moles per mole A reacted
Equations for the concentration
C_A = \frac{N_A}{V} = \frac{N_{A0}(1-X)}{V}
C_B = \frac{N_B}{V} = \frac{N_{B0}-(b/a)N_{A0}X}{V}
C_C = \frac{N_C}{V} = \frac{N_{C0}+(c/a)N_{A0}X}{V}
C_D = \frac{N_D}{V} = \frac{N_{D0}+(d/a)N_{A0}X}{V}
For a constant volume batch reactor, V = V_0.
Let \Theta_i= N_{i0}/N_{A0} = C_{i0}/C_{A0}.
- C_A = C_{A0}(1-X)
- C_B = C_{A0}(\Theta_B-(b/a)X)
- C_C = C_{A0}(\Theta_C+(c/a)X)
- C_D = C_{A0}(\Theta_D+(d/a)X)
\Theta_i= \frac{\text{Moles of species 'i' initially}}{\text{Moles of species A initially}}
Equimolar: \Theta_B = 1
Stoichiometric: \Theta_B = b/a
Rate expression
-r_A = k C_A C_B
-r_A = k C_{A0}^2 (1-X) (\phi_B-(b/a)X)
For C_{A0}= C_{B0}, and b/a = 1
-r_A = k C_{A0}^2 (1-X)^2
Mole balance
\frac{dC_A}{dt}=-k C_{A0}^2 (1-X)^2
as C_A = C_{A0}(1-X); dC_A = -C_{A0} dX
The mole balance can then be written as
\frac{dX}{(1-X)^2}=k C_{A0} dt
In class exercise: equilibrium conversion in batch reactor
Consider the following elementary reaction with K_C = 20 dm^3/mol and C_{A0} = 0.2 mol/dm^3. Find X_e for a batch reactor.
Reaction: \ce{2A <=> B}
Rate law: -r_A = k_A \left[ C_A^2 - \frac{C_B}{K_C} \right]
Flow systems
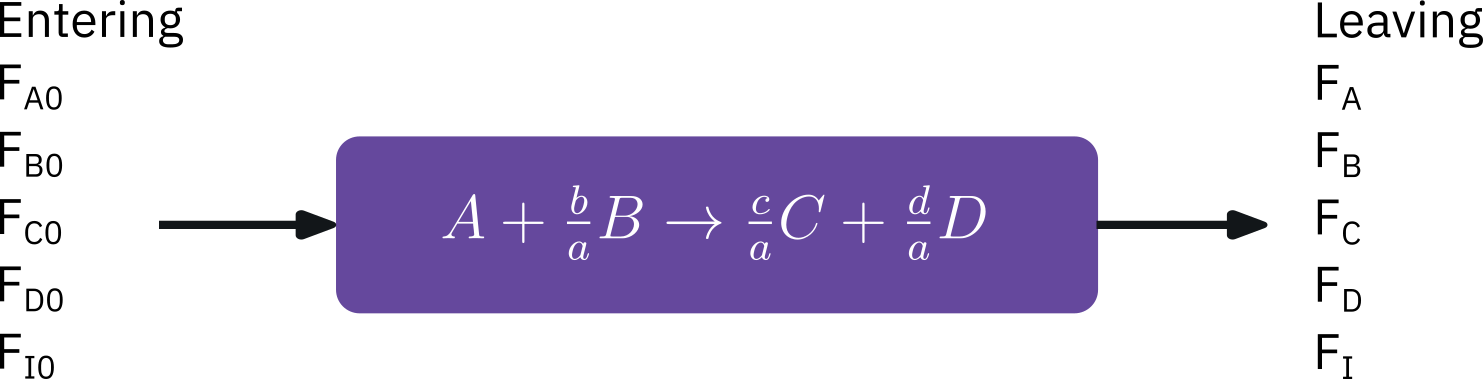
Form of stoichiometric table is virtually identical to batch systems
Replace N_{j0} by F_{j0}
Replace N_{j} by F_{j}
Stoichiometric table: flow systems
| Species | Feed rate to reactor (mol/time) | Change within reactor (mol/time) | Effluent rate from reactor (mol/time) |
|---|---|---|---|
| A | F_{A0} | -F_{A0}X | F_A = F_{A0} - F_{A0}X |
| B | F_{B0} | -(b/a)F_{A0}X | F_B = F_{B0} -(b/a)F_{A0}X |
| C | F_{C0} | (c/a)F_{A0}X | F_C = F_{C0} +(c/a)F_{A0}X |
| D | F_{D0} | (d/a)F_{A0}X | F_D = F_{D0} +(d/a)F_{A0}X |
| I | F_{I0} | 0 | F_I = F_{I0} |
| Total | \mathbf{F_{T0}} | \mathbf{F_T = F_{T0} + \delta F_{A0}X} |
\delta = +\frac{d}{a}+\frac{c}{a}-\frac{b}{a}-1
\Theta_{i0}= F_{i0}/F_{A0} = C_{i0}/C_{A0}.
C_B = C_{A0}(\Theta_B-(b/a)X)
For liquid phase systems (\upsilon = \upsilon_0)
Gas phase reactions
Volumetric flow rate changes during the course of reaction
Changes in total number of moles
Changes in pressure and temperature
Variable flow rate
Gas phase reactions that do not have equal number of product and reactant moles
e.g. \ce{N2 + 3H2 <=> 2NH3}
- 4 mole reactant give 2 mole products
Stoichiometric tables
No assumptions made reagarding volume
The tables are exactly same for constant volume (constant density) and variable volume (variable density) systems
\Rightarrow Only for concentration expressed in terms of conversion density/ volume comes into play.
Flow reactor with variable volumetric flow rate
We will use relationships for total concentration
C_T = \frac{F_T}{\upsilon} \qquad ... \qquad \frac{\text{total molar flow rate}}{\text{volumetric flow rate}}
For gases
C_T = \frac{P}{zRT} \qquad z: \text{compressibility factor (=1 for ideal gas)}
At the entrance of the reactor
C_{T0} = \frac{P_0}{z_0RT_0}
Assuming negligible change in z (z = z_0)
\upsilon = \upsilon_0 \left( \frac{F_T}{F_{T_0}} \right) \left( \frac{P_0}{P} \right) \left( \frac{T}{T_0} \right)
Flow reactor with variable volumetric flow rate
We can now express C_j (=F_j/\upsilon) in terms of F, P, and T
C_j = C_{T0} \left( \frac{F_j}{F_T} \right) \left( \frac{P}{P_0} \right) \left( \frac{T_0}{T} \right)
F_T = \sum_{j=1}^{n} F_j = F_A + F_B + F_C + F_D + F_I
F_j is found by solving mole balance equations
Concentration in terms of conversion: F_T = F_{T0} + F_{A0} \delta X
Dividing by F_{T0} and defining \epsilon = y_{A0} \delta, where y_{A0} is the mole fraction of A at inlet
\frac{F_T}{F_{T0}} = 1 + \epsilon X
\epsilon = \frac{ \text{change in total no. of moles for complete conversion}} { \text{total moles fed}}
Flow reactor with variable volumetric flow rate
Equation for volumetric flow rate \upsilon = \upsilon_0 (1 + \epsilon X) \left( \frac{P_0}{P} \right) \left( \frac{T}{T_0} \right)
Molar flow rate F_j = F_{A0} (\Theta_j + \nu_j X)
Concentration (C_j = F_j /\upsilon)
C_j = \frac{F_{A0} (\Theta_j + \nu_j X)} {\upsilon_0 (1 + \epsilon X) \left( \frac{P_0}{P} \right) \left( \frac{T}{T_0} \right) }
- Stoichiometric coefficient (\nu_j)
\ce{ A + \frac{b}{a} B -> \frac{c}{a} C + \frac{d}{a} D}
-ve for reactant
\nu_A = -1; \nu_B = -b/a
+ve for products
\nu_C = c/a; \nu_D = -d/a
C_j = \frac{C_{A0} (\Theta_j + \nu_j X)} {(1 + \epsilon X)} \left( \frac{P}{P_0} \right) \left( \frac{T_0}{T} \right)
In class exercise: equilibrium conversion in flow reactor
Consider the following elementary reaction with K_C = 20 dm^3/mol and C_{A0} = 0.2 mol/dm^3. Find X_e for a flow reactor.
Reaction: \ce{2A <=> B}
Rate law: -r_A = k_A \left[ C_A^2 - \frac{C_B}{K_C} \right]
In class exercise: saponification reaction
The saponification for the formation of soap is:
\ce{3NaOH + (C17H35COO)3C3H5 -> 3C17H35COONa + C3H5(OH)3}
Letting X represent the conversion of NaOH set up a stoichiometric table expressing the concentration of each species in terms of the initial concentration of NaOH and the conversion of X.
In class exercise: determining C_j = h_j(X)
A mixture Of 28% \ce{SO2} and 72% air is charged to a flow reactor in which \ce{SO2} is oxidized.
\ce{2SO2 + O2 -> 2 SO3}
First, set up a stoichiometric table using only the symbols (i.e., \Theta_i, F_i).
Next, prepare a second table evaluating the species concentrations as a function of conversion for the case when the total pressure is 1485 kPa (14.7 atm) and the temperature is constant at 227 °C.
Evaluate the parameters and make a plot of each of the concentrations \ce{SO2}, \ce{SO3}, \ce{N2} as a function of conversion
In class exercise: liquid phase first order reaction
Orthonitroanaline (an important intermediate in dyes—called fast orange) is formed from the reaction of orthonitrochlorobenzene (ONCB) and aqueous ammonia. The liquid-phase reaction is first order in both ONCB and ammonia with k = 0.0017 \ m^3 /kmol \cdot min at 188 \ ^{\circ}C with E = 11273 \ cal/mol. The initial entering concentrations of ONCB and ammonia are 1.8 \ kmol/m^3 and 6.6 \ kmol/m^3, respectively.
\ce{C6H4ClNO2 + 2 NH3 -> C6H6N2O2 + NH4Cl}
Set up a stoichiometric table for this reaction for a flow system.
Write the rate law for the rate of disappearance of ONCB in terms of concentration.
Explain how parts (a) and (b) would be different for a batch system.
Write -r_A solely as a function of conversion.
What is the initial rate of reaction (X = 0) at 188 \ ^{\circ}C, at 25 \ ^{\circ}C, and at 288 \ ^{\circ}C?
What is the rate of reaction when X = 0.90 at 188 \ ^{\circ}C, at 25 \ ^{\circ}C, and at 288 \ ^{\circ}C?
What would be the corresponding CSTR reactor volume at 25 \ ^{\circ}C to achieve 90% conversion and at 288 \ ^{\circ}C for a feed rate of 2 \ dm^3 /min
Summary
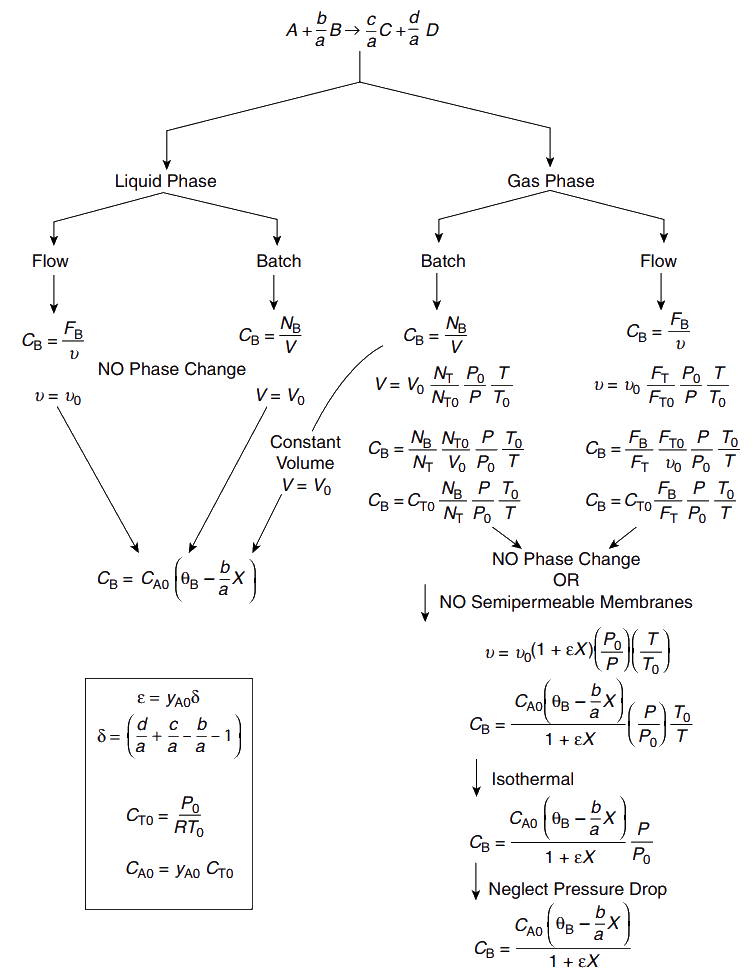
Fogler, H. Scott. 2016. Elements of Chemical Reaction Engineering. Fifth edition
Chemical Reaction Engineering